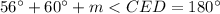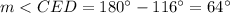Answer:
Part a)
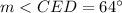
Part b)
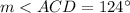
Explanation:
step 1
Find the measure of angle ACB
we know that
The sum of the measures of interior angle of a triangle must be equal to
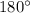
so
In the triangle ABC
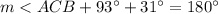
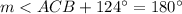
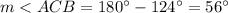
step 2
Find the measure of angle ACD
we know that
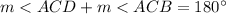 -------> by supplementary angles
-------> by supplementary angles
we have
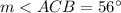
substitute

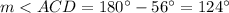
step 3
Find the measure of angle CED
we know that
m<DCE=m<ACB ------> by vertical angles
so

Remember that
The sum of the measures of interior angle of a triangle must be equal to
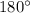
so
In the triangle CDE