Question

The state fair is a popular field trip destination. This year the senior class at High School A and the senior class at High School B both planned trips there. The senior class at High School A rented and filled 1 van and 10 buses with 258 students. High School B rented and filled 8 vans and 5 buses with 264 students. Each van and each bus carried the same number of students. How many students can a van carry? How many students can a bus carry?

Answer 1

Answer: There are 18 students in a van and 24 students in a bus.

Explanation:

Let the number of students in the van be 'x' and the number of students in bus be 'y'.

Then, According to the question, we have the following system

`\text{[High School A ]}\ x+10y=258........................(1)\\\\\text{[High School B ]}\ 8x+5y=264........................................(2)\\`

Multiply 2 to both the sides of equation (2), we get

`16x+10y=528.......................(3)`

Subtract equation (1) from equation (3), we get

`15x=270\\\Rightarrow\ x=(270)/(15)\\\Rightarrow\ x=18`

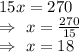
Substitute value of x in (1), we get

`18+10y=258\\\Rightarrow\ 10y=240\\\Rightarrow\ y=24`

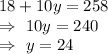
Hence, there are 18 students in a van and 24 students in a bus.