Suppose a triangle had two sides of length 2 and 3 and that the angle between there two sides is 60. what is the length of the third side of the triangle

Question

asked Oct 12, 2020 110k views

1 Answer

Zahan Safallwa · Answer 1 · 2020-10-17T10:56:06+0000

Answer:

The length of third sides of the triangle is 2.65 units.

Explanation:

It is given that triangle had two sides of length 2 and 3 and that the angle between there two sides is 60.

a = 2 units, b = 3 units and angle C = 60°.

Law of Cosine:

$c^2=a^2+b^2-2ab\cos C$

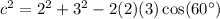
$c^2=2^2+3^2-2(2)(3)\cos (60^(\circ))$

c^2=4+9-12((1)/(2))

c^2=7

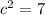
Square root both sides.

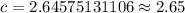
$c=2.64575131106\approx 2.65$

Therefore the length of third sides of the triangle is 2.65 units.