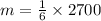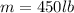ANSWER
450lb
Step-by-step explanation
If the weight, m of an object on the moon varies directly as the weight of an object e, on the earth, then we can write the mathematical statement,.
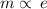
We introduce the constant of proportionality to obtain,
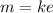
When, m=95, e=570,
This implies that,
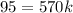

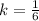
The equation now becomes,
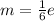
We want to find e, when m=2700,