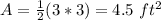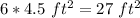Question

Plz help on both will give branlyist and thx

Answer 1

Part 1)
`132\ ft^(2)`

Part 2)
`27\ ft^(2)`

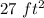
Explanation:

Part 1) we know that

The area of the figure is the area of one trapezoid multiplied by 2

Find the area of one trapezoid

`A=(1)/(2)(b1+b2)h`

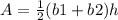
we have

`b1=9\ ft`

`b2=15\ ft`

`h=11/2=5.5\ ft`

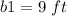
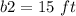
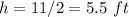
substitute

`A=(1)/(2)(9+15)5.5=66\ ft^(2)`

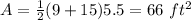
Find the area of the figure

`2*66\ ft^(2)=132\ ft^(2)`

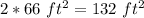
Alternative Method

The area of the figure is equal to the area of a rectangle plus the area of triangle

so

`A=11*9+(1)/(2)(11)(15-9)\\ \\A=99+33\\\\A=132\ ft^(2)`

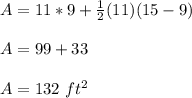
Part 2) we know that

The area of the hexagon is equal to the area of one triangle multiplied by 6

Find the area of one triangle

`A=(1)/(2)bh`

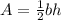
we have

`b=3\ ft`

`h=3\ ft`

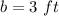
substitute

`A=(1)/(2)(3*3)=4.5\ ft^(2)`

`6*4.5\ ft^(2)=27\ ft^(2)`