Question

Select the correct answer. Which point lies on a circle with a radius of 5 units and center at P(6, 1)? A. Q(1, 11) B. R(2, 4) C. S(4, -4) D. T(9, -2)

Answer 1

T(9,-2)

Explanation:

The circle has radius 5 units and center P(6,1).

The equation of this circle is

`(x-6)^2+(y-1)^2=5^2`

`(x-6)^2+(y-1)^2=25`

If Q(1,11) lies on this circle, then it must satisfy its equation.

`(1-6)^2+(11-1)^2=25`

`(-5)^2+(10)^2=25`

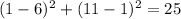
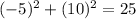
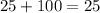`25+100=25`, this statement is false.

If R(2,4) lies on this circle, then it must satisfy its equation.

`(2-6)^2+(4-1)^2=25`

`(-4)^2+(3)^2=25`

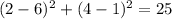
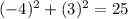
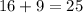`16+9=25`, this statement is false.

If S(4,-4) lies on this circle, then it must satisfy its equation.

`(4-6)^2+(-4-1)^2=25`

`(-2)^2+(-5)^2=25`

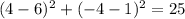
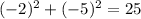
`4+25=25`, this statement is false.

If T(9,-2) lies on this circle, then it must satisfy its equation.

`(9-6)^2+(-2-1)^2=25`

`(4)^2+(-3)^2=25`

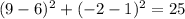
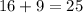`16+9=25`, this statement is TRUE.

The correct answer is D

Answer 2

Option B)

R(2,4)

Explanation:

Given that P(6,1) is the center of the circle.

Let us find each point distance from P if equal to 5 units, then the point lies on the circle

PQ =
`√((1-6)^2+(11-1)^2) =√(125) \\=5√(3)`

PR =
`√((2-6)^2+(4-1)^2) =√(25) =5`

PS =
`√((4-6)^2+(-4-1)^2) \\=√(29)`

PT =
`√((9-6)^2+(-2-1)^2) \\=√(18) \\=3√(2)`

We see that only R is having a distance of 5 units from P.

Hence R lies on the circle

Option B)

R(2,4)