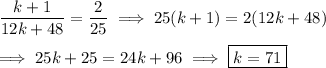(a.i) If
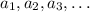 are in arithmetic progression, then there is a constant
are in arithmetic progression, then there is a constant
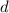 such that
such that
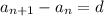
for all
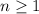 . In other words, the difference
. In other words, the difference
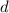 between any two consecutive terms in the sequence is always the same.
between any two consecutive terms in the sequence is always the same.
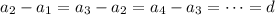
Now, we can expand the target expression into partial fractions.
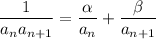
Combining the fractions on the right and using the recursive equation above, we have
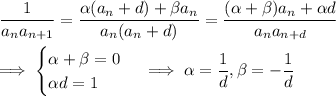
and hence
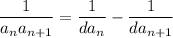
as required.
(a.ii) Using the previous result, the
 -th term
-th term
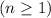 in the sum on the left is
in the sum on the left is
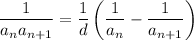
Expand each term in this way to reveal a telescoping sum:

By substitution, we can show

so that the last expression reduces to
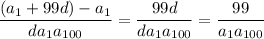
as required. More generally, it's easy to see that
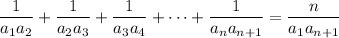
(b) I assume you mean the equation
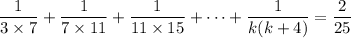
Note that the distinct factors of each denominator on the left form an arithmetic sequence.
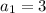
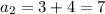
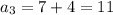
and so on, with
 -th term
-th term
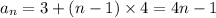
Let
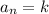 . Using the previous general result, the left side reduces to
. Using the previous general result, the left side reduces to
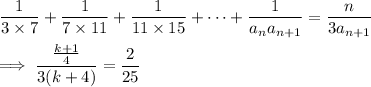
Solve for
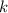 .
.