Answer:
The coordinates of point D is (1, 0)
Explanation:
Given that points A(-2,4), B(1, 3), C(4, -1) and D form a parallelogram.
we have to find the coordinates of point D.
Let coordinates of point D are (x, y)
By mid-point formula, if (a, b) and (c, d) are the coordinates of two points joining the line segment then the coordinates of mid-point are
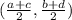
As diagonals of parallelogram bisect each other therefore the mid-point of both diagonals are same.
Mid-point of AC=Mid-point of BD
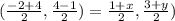
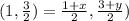
Comparing both sides, we get
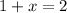 ⇒
⇒

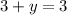 ⇒
⇒

The coordinates of point D is (1, 0)
Option D is correct