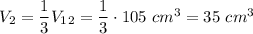Question

A cylinder has a volume of 105 cubic centimeter what is the volume of a cone with the same base and height?

315cm3
210cm3
70cm3
35cm3

Answer 1

35 cm³

Explanation:

The formula of a volume of a cylinder:

`V_1=\pi r^2H`

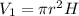
r - radius

H - height

The formula of a volume of a cone:

`V_2=(1)/(3)\pi r^2H`

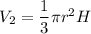
If the cylinder and the cone have the same base (radius) and the same height, then the volume of the cone is three times smaller than the volume of the cylinder.

`V_2=(1)/(3)\underbrace{\pi r^2H}_(V_1)`

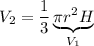
Therefore:

`V_2=(1)/(3)V_1\toV_2=(1)/(3)\cdot105\ cm^3=35\ cm^3`