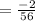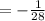Answer:
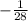
Explanation:
The given function is
![f(x)=-\sqrt[3]{x}](https://img.qammunity.org/2020/formulas/mathematics/college/5diy0n3er8p153s8ntfm2gwu5jkifwx8td.png)
![f(64)=-\sqrt[3]{64}=-4](https://img.qammunity.org/2020/formulas/mathematics/college/4a0jggikdib6t4didvy8dsgftatksclmx8.png)
![f(8)=-\sqrt[3]{8}=-2](https://img.qammunity.org/2020/formulas/mathematics/college/alzpfz8wxxx43kqtcwd4k8l4cc71c4zyk2.png)
The average rate of change of this function is the slope of the secant line connecting the points (64,f(64)) and (8,f(8));

We substitute the functional values into this function to obtain;
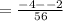
This simplifies to;