Question

Find the quotient. 12 a3 p4 ÷ -2a2p

Answer 1

-6a / p³

Explanation:

Given expression is

`12a^(3) p^(4) /(-2a^(2)p)`

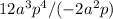
We have to simplify above expression.

We use two formulas in this expression.

xⁿ = 1/x⁻ⁿ (formula 1)

`x^(m)`×
`x^(n)` =
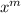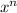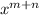`x^(m+n)` ( formula 2)

using (formula 1) in given expression,we get

`12 a^(3) p^(4) a^(-2) p^(-1) /-2`

using (formula 2 ) in above expression ,we get

`12a^(3-2) p^(4-1) /-2`

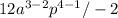
12a¹p⁻³ / -2

2×6ap⁻³ / -2

-6a / p³ which is the answer.

Answer 2

Answer:
`-6ap^3`

Explanation:

Here the given expression is,

`(12a^3p^4)/(-2a^2p)`

=
`(-12* a^3* a^(-2)* p^4* p^(-1))/(2)`
`( (1)/(a^x) = a^(-x) )`

=
`-6* a^(3-2)* p^(4-1)`
`( a^m* a^n = a^(m+n) )`

=
`-6 * a^1* p^3`

=
`-6ap^3`