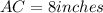Answer:
Explanation:
It is given that triangle AOC intersects a circle with center O, side AO is 10 inches and the diameter of the circle is 12 inches, thus
OC is the radius of the circle and is equal to
 .
.
Now, From ΔAOC, using the Pythagoras theorem, we get
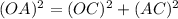
⇒
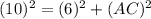
⇒
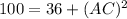
⇒
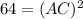
⇒