Answer: The answer is -1.
Step-by-step explanation: Given that 'a' and 'b' are integers such that their product is 5. We are to find the least possible value of 'a to the power b'.
Since a and b are integers and a × b = 5, so their possible values are
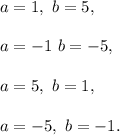
For these only four choices of a and b, we have the following values of a to the power b:

since -1 is the smallest among these four values, so the least possible value of 'a to the power b' is -1.
Thus, the answer is -1.