Question

Use the formula to evaluate the series 1+2+4+8+...a10

Answer 1

The sum of the given series is 1023

Explanation:

Geometric series states that a series in which a constant ratio is obtained by multiplying the previous term.

Sum of the geometric series is given by:

`S_n = (a(1-r^n))/(1-r)`

where a is the first term and n is the number of term.

Given the series:
`1+2+4+8+.................+a_(10)`

This is a geometric series with common ratio(r) = 2

We have to find the sum of the series for 10th term.

⇒ n = 10 and a = 1

then;

`S_n = (1(1-2^(10)))/(1-2) =(1-1024)/(-1) =(-1023)/(-1)=1023`

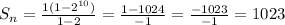
Therefore, the sum of the given series is 1023