Answer:
A) f(x): y-intercept is
 and g(x): y-intercept is (0,0)
and g(x): y-intercept is (0,0)
B) f(x): asymptote is x= 0 and g(x): asymptote is x= 4.
Explanation:
We are given the functions,
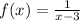 and g(x) graphed.
and g(x) graphed.
A): We know that, 'y-intercepts are the points where the graph of the function cuts y-axis'
That is, 'y-intercepts are obtained when x= 0'.
So, we have,
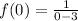 i.e.
i.e.
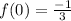 .
.
Thus, the y-intercept of the function f(x) is the point
 .
.
Furhter, from the graph of g(x), we see that,
The graph of the function g(x) crosses y-axis at the point (0,0).
Thus, the y-intercept of the function g(x) is the point (0,0).
B): We know that, 'asymptotes are the lines that approaches the curves but does not meet them'.
Since, the function
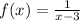 has a numerator of lower degree than the denominator.
has a numerator of lower degree than the denominator.
Thus, x= 0 is the horizontal asymptote of the function f(x).
Also, from the graph of g(x), we see that, The line x= 4 is approaching the curve infinitely.
Thus, the vertical line x= 4 is the asymptote of the function g(x).