Answer: 20 ft
This problem can be solved by the Thales’s theorem, which states:
Two triangles are similar when they have equal angles and proportional sides
It should be noted that to apply Thales' Theorem, it is necessary to establish the two triangles are similar, that is, that they have the corresponding angles equal or that their sides are proportional to each other.
Now, if we measure the shadow of the flagpole and the shadow of the person, at the same moment, we can use the first Thales' Theorem to calculate the height of the flagpole, knowing the height of the person.
In this case we have two similar triangles (Figure attached) where
 is the height of the flagpole,
is the height of the flagpole,
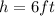 is the height of the person,
is the height of the person,
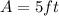 is the length of the shadow of the flagpole and
is the length of the shadow of the flagpole and
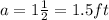 is the length of the shadow of the person.
is the length of the shadow of the person.
Having this clear, we can write the following relation with both similar triangles:
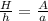 (1)
(1)
We know all these lengths except
 , which is the value we want to to find.
, which is the value we want to to find.
So, in order to approach this problem we have to find
 from equation (1):
from equation (1):
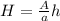
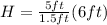
Then:
 >>>>>This is the height of the flagpole
>>>>>This is the height of the flagpole