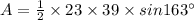Question

In triangle PQR, PR = 23mm, QR = 39mm, and m<R = 163 degrees. Find the area of the triangle to the nearest tenth.

Answer 1

Answer: 131.1287 square mm (approx)

Explanation:

The area of a triangle,

`A=(1)/(2) * s_1* s_2* sin \theta`

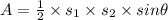
Where
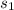`s_1` and
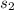`s_2` are adjacent sides and
`\theta` is the include angle of these sides,

Here PR and QR are adjacent sides and ∠R is the included angle of these sides,

Thus, we can write,

`s_1 = PR= 23\text{ mm}`,
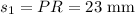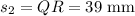`s_2=QR=39\text{ mm}` and
`\theta = 163^(\circ)`,

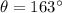Thus, the area of the triangle PQR,

`A=(1)/(2) * 23* 39* sin163^(\circ)`

`A=(262.257419136)/(2) = 131.128709568\approx 131.1287\text{ square mm}`