Answer:
1.
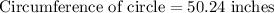
2.
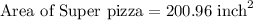
3.

4. Area.
Explanation:
We have been given that Mr. Bernard needs to order more boxes for his 16 inch diameter “Super Pizza”.
1. Since we know that circumference of a circle equals to
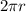 , where r represents radius of the circle.
, where r represents radius of the circle.
To find the circumference of our given pizza we need to find radius of the pizza. Since we know that diameter of a circle is 2 times the radius, so dividing the diameter of Super pizza by 2 we will get our radius.
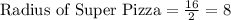
Upon substituting our given values in circumference formula we will get,

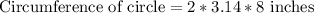
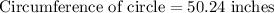
Therefore, circumference of Super pizza is 50.24 inches.
2. Since we know that area of circle equals to
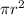 .
.
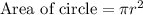
Upon substituting r=8 in area formula we will get,

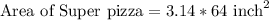
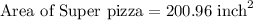
Therefore, area of super pizza in 200.96 square inches.
3. To find the total cost of pizza we will multiply the area of pizza by cost of per square inch pizza.
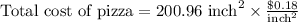


Therefore, the total cost of pizza will be $36.17.
4. We can see that area of Super pizza is 200.96 square inches, while circumference of Super pizza is 50.24 inches. As 200.96 is greater than 50.24, therefore, area of the pizza is greater.