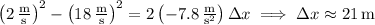Use Newton's second law to determine the acceleration applied by the stopping force:

Then recall that

Take the direction in which the car is traveling to be positive, so that its acceleration points in the opposite direction and
 has negative sign. Then
has negative sign. Then