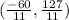Question

Given A(-12,5) and B(12,29) find the point that divides the line segment AB three-eighths of the way from A to B

Answer 1

`\text{The coordinates are}((-60)/(11),(127)/(11))`

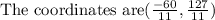
Explanation:

Given two points A(-12,5) and B(12,29). We have to find the point that divides the line segment AB three-eighths of the way from A to B.

By section formula, when a point C divides a segment AB in the ratio m:n, then the coordinates of point C are

`C(((mx_2+nx_1))/((m+n)),((my_2+ny_1))/((m+n)))`

⇒
`C(((3(12)+8(-12)))/((3+8)),((3(29)+8(5)))/((3+8)))`

⇒
`C(((36-96))/(11)),((87+40))/((11)))`

⇒
`C((-60)/(11),(127)/(11))`

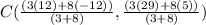
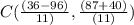
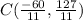
Hence, the coordinates of point C that divides the line segment AB three eighths of the way from A to B are
`((-60)/(11),(127)/(11))`