Answer:
600 daylilies were on the farm after the first year.
Explanation:
Given : A daylily farm sells a portion of their daylilies and allows a portion to grow and divide. The recursive formula
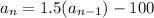 represents the number of daylilies, a, after n years. After the fifth year, the farmers estimate they have 2225 daylilies.
represents the number of daylilies, a, after n years. After the fifth year, the farmers estimate they have 2225 daylilies.
To find : How many daylilies were on the farm after the first year?
Solution :
The recursive formula is
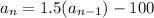
We have given after the fifth years number of daylilies,
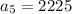
Put n=5 in the formula we get,
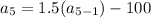
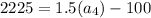
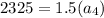
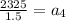
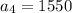
Now, put n=4 in the formula,

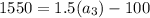

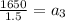
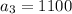
Now, put n=3 in the formula,
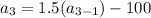
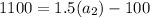
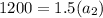

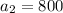
Now, put n=2 in the formula,
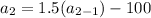
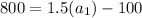
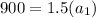
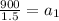
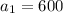
Which means after first year is
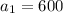
Therefore, 600 daylilies were on the farm after the first year.