Question

In trapezoid WXYZ, identify WX. PLEASE HELP!!!

Answer 1

The length of WX is 31 units.(third option)

Explanation:

Given that In trapezoid WXYZ, WX║ZY and S and T are the mid points of the sides WZ and XY. We have to find the length of WX.

By trapezoid mid-segment Property,

A mid-segment of a trapezoid join the midpoints of the two non-parallel sides of trapezoid. The length of the mid-segment is the average of the lengths of the two bases and is parallel to the bases of the trapezoid.

∴
`ST=(WX+ZY)/(2)`

⇒
`48=(WX+65)/(2)`

⇒
`96=WX+65`

⇒
`WX=96-65=31`

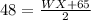
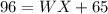
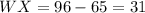
The length of WX is 31 units.(third option)