Question

A lake has a small population of a rare endangered fish. The lake currently has a population of 10 fish. The number of fish is increasing at a rate of 4% per year. When will the population double? How long will it take the population to be 80 fish?

Answer 1

Answer: 1) After 17.673 years ( Approx) the population will be doubled.

2) After 53.019 years the population will be 80.

Explanation:

1) Let x represents the number of years after which the population will doubled,

Since rate of interest = 4%

And, initial number of fish = 10,

Hence the fish after x years =
`10(1+(4)/(100))^x =10(1+0.04)^x =10(1.04)^x`

⇒
`10(1.04)^x = 20`

⇒
`(1.04)^x = 2`

⇒
`x log (1.04) = log(2)`

⇒
`x = (log(2))/(log(1.04))=17.6729877\approx 17.673`

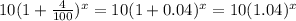
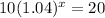
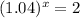
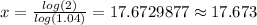
Hence, After 17.673 years ( Approx) the population will be doubled.

2) Let after y years the population will be 80.

⇒
`10(1.04)^x = 80`

⇒
`(1.04)^x = 8`

⇒
`x log (1.04) = log(8)`

⇒
`x = (log(8))/(log(1.04))=53.0189631\approx 53.019`

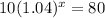
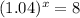
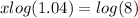
Hence, After 53.019 years the population will be 80.