Answer:
The average rate of change of f(x) from x = a to x = a + h is -1
Explanation:
Average rate of the function f(x) over [a, b] is given by:
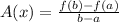
Given the function:
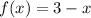
At x=a
f(a) = 3-a
At x = a+h
then;
f(a+h) = 3-(a+h) = 3-a-h
Using the formula for average rate of function:
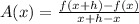
Substitute the given values we have;
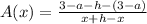
Simplify:

or
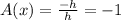
Therefore, the average rate of change of f(x) from x = a to x = a + h is, -1