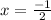Question

What are the solutions of the equation?

6x^2 + 11x + 4 = 0

a. 4/3, 1/2
b. -4/3, -1/2
c. 4/3, -1/2
d. -4/3, 1/2

Answer 1

b. -4/3, -1/2

Explanation:

We are given the following equation and we are to solve it by factorizing it:

`6x^2 + 11x + 4 = 0`

We are to find factors of 24 such that when multiplied they give a product of 24 and when added they give a result of 11.

`6x^2+3x+8x+4=0`

`3x(2x+1)+4(2x+1)=0`

`(2x+1)(3x+4)=0`

`2x+1=0, 3x+4=0`

`x=-(1)/(2) , x=-(4)/(3)`

Therefore, the correct answer option is b. -4/3, -1/2.

Answer 2

Correct option is b;
`x= (-4)/(3),(-1)/(2)`

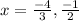
Explanation:

Given equation 6x² + 11x + 4 = 0

Using factorization method to solve it

6x² + 8x + 3x + 4 = 0

Dividing the central term into two terms in such a way that their product gives 24x² and their sum gives 11x

taking common in the above equation

2x(3x+4) + 1(3x+4) = 0

taking 3x+4 common

(3x+4)(2x+1) = 0 --------------eq 1

we can write from eq1

(3x+4) = 0

3x = -4

`x= (-4)/(3)`

and we can write from eq1

2x+1 = 0

2x = -1

`x= (-1)/(2)`