Question

a rectangle is 8cm longer then it is wide, and it’s area is 153cm^2. Find the dimensions of the rectangle.

Answer 1

Width of 9cm and length of 17cm.

Explanation:

To calculate an area of a rectangle, use the formula A=l*w. We know the length is 8cm longer than the width. So l = 8 + w. So the area is A= (8+w)*w.

We also know the area is 153. Substitute this value for A and solve.

`153 = (8+w)(w)\\153 = 8w+w^2\\w^2+8w-153 = 0`

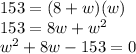
To solve the quadratic, use the quadratic formula:

`(-b+/-√(b^2-4ac) )/(2a)`

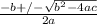
Here a=1, b=8 and c=-153.

`w=(-b+/-√(b^2-4ac) )/(2a) \\w=(-8+/-√(8^2-4(1)(-153)) )/(2(1)) \\w=(-8+/-√(64+612) )/(2) \\w=(-8+/-√(676) )/(2) \\w=(-8+/-26 )/(2) \\w=(-8+26 )/(2)=(18)/(2)=9 \\and\\w=(-8-26 )/(2) =(-34)/(2)=-17`

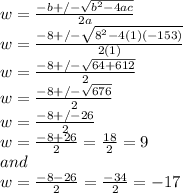
Since w=9 or w=-17, substitute this value for w in l= 8+w to find l.

l = 8+9 = 17

or

l=8+-17 = -9

Since length cannot be positive, it must be l=17 and w=9.