Question

Find the inverse of each function for problems 1–6. State the domain and range of both the function and its inverse. Restrict the domain of the function if needed.

1. f(x) = –x2

2. f(x)=5x–1

3. f(x)=–x+3

4. f(x)=x2+7

5. f(x)=14x−4

6. f(x)=–3x+8

Answer 1

1.

Function:

`f(x)=-x^2`

Domain: (-∞,∞)

Range: (-∞,0]

Inverse Function:

`f^(-1)(x)=√(-x) ,and\\f^(-1)(x)=-√(-x)`

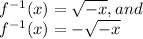
Domain: (-∞,0]

Range: (-∞,∞)

2.

Function:

`f(x)=5x-1`

Domain: (-∞,∞)

Range: (-∞,∞)

Inverse Function:

`f^(-1)(x)=(1)/(5)x+(1)/(5)`

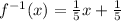
Domain: (-∞,∞)

Range: (-∞,∞)

3.

Function:

`f(x)=-x+3`

Domain: (-∞,∞)

Range: (-∞,∞)

Inverse Function:

`f^(-1)(x)=-x+3`

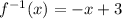
Domain: (-∞,∞)

Range: (-∞,∞)

4.

Function:

`f(x)=x^(2)+7`

Domain: (-∞,∞)

Range: [7,∞)

Inverse Function:

`f^(-1)(x)=√(x-7), and\\f^(-1)(x)=-√(x-7)`

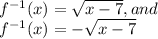
Domain: [7,∞)

Range: (-∞,∞)

5.

Function:

`f(x)=14x-4`

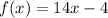
Domain: (-∞,∞)

Range: (-∞,∞)

Inverse Function:

`f^(-1)(x)=(1)/(14)x+(2)/(7)`

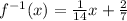
Domain: (-∞,∞)

Range: (-∞,∞)

6.

Function:

`f(x)=-3x+8`

Domain: (-∞,∞)

Range: (-∞,∞)

Inverse Function:

`f^(-1)(x)=-(1)/(3)x+(8)/(3)`

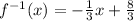
Domain: (-∞,∞)

Range: (-∞,∞)

Explanation:

To find inverse of a function f(x), there are 4 steps we need to follow:

1. Replace f(x) with y

2. Interchange the y and x

3. Solve for the "new" y

4. Replace the "new" y with the notation for inverse function,
`f^(-1)(x)`

Note: The domain of the original function f(x) is the range of the inverse and the range of the original function is the domain of the inverse function.

Let's calculate each of these.

1.

`f(x)=-x^2`

Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: No matter what we put into x, the y values will always be negative. And if we put 0, y value would be 0. So range is (-∞,0]

Finding the inverse:

`f(x)=-x^2\\y=-x^2\\x=-y^2\\y^2=-x\\y=+-√(-x) \\y=√(-x), -√(-x)`

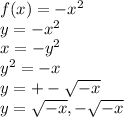
So

`f^(-1)(x)=√(-x) ,and\\f^(-1)(x)=-√(-x)`

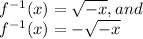
Domain: this is the range of the original so domain is (-∞,0]

Range: this is the domain of the original so range is (-∞,∞)

2.

`f(x)=5x-1`

Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: All sorts of y values will occur, so the range is (-∞,∞)

Finding the inverse:

`f(x)=5x-1\\y=5x-1\\x=5y-1\\5y=x+1\\y=(1)/(5)x+(1)/(5)`

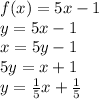
So

`f^(-1)(x)=(1)/(5)x+(1)/(5)`

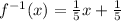
Domain: this is the range of the original so domain is (-∞,∞)

Range: this is the domain of the original so range is (-∞,∞)

3.

`f(x)=-x+3`

Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: All sorts of y values will occur, so the range is (-∞,∞)

Finding the inverse:

`f(x)=-x+3\\y=-x+3\\x=-y+3\\y=-x+3`

So

`f^(-1)(x)=-x+3`

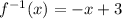
Domain: this is the range of the original so domain is (-∞,∞)

Range: this is the domain of the original so range is (-∞,∞)

4.

`f(x)=x^(2)+7`

Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: no matter what we put into x, it will always be a positive number greater than 7. Only when we put in 0, y will be 7. So 7 is the lowest number and it can go to infinity. Hence the range is [7,∞)

Finding the inverse:

`f(x)=x^2+7\\y=x^2+7\\x=y^2+7\\y^2=x-7\\y=+-√(x-7)`

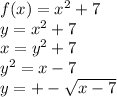
So

`f^(-1)(x)=√(x-7), and\\f^(-1)(x)=-√(x-7)`

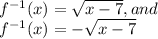
Domain: this is the range of the original so domain is [7,∞)

Range: this is the domain of the original so range is (-∞,∞)

5.

`f(x)=14x-4`

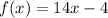
Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: no matter what we put into x, we can get any y value from negative infinity to positive infinity. So range is (-∞,∞)

Finding the inverse:

`f(x)=14x-4\\y=14x-4\\x=14y-4\\14y=x+4\\y=(1)/(14)x+(2)/(7)`

So

`f^(-1)(x)=(1)/(14)x+(2)/(7)`

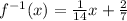
Domain: this is the range of the original so domain is (-∞,∞)

Range: this is the domain of the original so range is (-∞,∞)

6.

`f(x)=-3x+8`

Domain: There is no restriction on values of x we can put on it. Hence domain is (-∞,∞)

Range: no matter what we put into x, we can get any y value from negative infinity to positive infinity. So range is (-∞,∞)

Finding the inverse:

`f(x)=-3x+8\\y=-3x+8\\x=-3y+8\\3y=-x+8\\y=-(1)/(3)x+(8)/(3)`

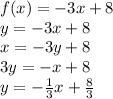
So

`f^(-1)(x)=-(1)/(3)x+(8)/(3)`

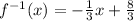
Domain: this is the range of the original so domain is (-∞,∞)

Range: this is the domain of the original so range is (-∞,∞)