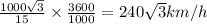Answer:

Explanation:
Let AB denote the height of eagle and and after 15 seconds it will become DE.
Also denote C is the point where boy is standing.
Now since both the triangles ABC and DEC are right angle triangle.
Now, in triangle ABC,
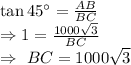
In triangle DEC,
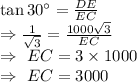
Now, EB=
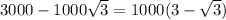
Speed of eagle =
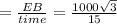
In km/hr
Speed of eagle =