Question

The radius of a 10 inch right circular cylinder is measured to be 4 inches, but with a possible error of ±0.1 inch. What is the resulting possible error in the volume of the cylinder? Include units in your answer

Answer 1

Possible error in the volume of the cylinder is ±8π inch³.

Explanation:

We have, a right circular cylinder with radius, r = 4 inches and height, h = 10 inches.

As, the volume of a cylinder =
`\pi r^(2) h`

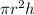
So, volume of the given cylinder, V =
`10\pi r^(2)`

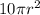
Then,
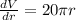`(dV)/(dr)=20\pi r` i.e.
`dV=(20\pi r)dr`,

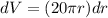where dV and dr represents the possible change (or error) in volume and radius of the cylinder respectively.

As, we have,

r = 4 inches and dr = ±0.1 inch.

Substituting the values, we get,

`dV=(20\pi r)dr`

⇒
`dV=20\pi 4* \pm 0.1`

⇒
`dV=80\pi * \pm 0.1`

⇒
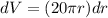
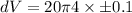
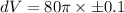
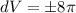`dV=\pm 8\pi` inch³

Hence, the possible error in the volume of the cylinder is ±8π inch³.