Answer:
correct answers are option 1 (Q and W are similar but not congruent) and option 4 (Q and S are similar but not congruent)
Explanation:
Congruent:
Two quadrilateral are said to be congruent if they have equal length and breadth and they have exactly same shape.
Similar:
Two quadrilaterals will said to be similar if their sides are proportional . It means ratio of their length is equal to the ratio of their breath then the quadrilateral will said to be similar.
From the given graph it is clear that each angle of quadrilaterals Q, S and W is equal to
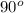 therefore these all quadrilaterals are rectangles.
therefore these all quadrilaterals are rectangles.
In graph it is given that,
The length of rectangle is
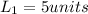
The length of rectangle Q is

Breadth of rectangle S
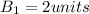
Breadth of rectangle Q
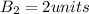
Therefore, it is clear that length and breadth of rectangle Q are equal to length and breadth of rectangle S and all the angles of Q and S are equal to
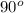 therefore, quadrilaterals Q and S are similar and congruent.
therefore, quadrilaterals Q and S are similar and congruent.
From graph it is given,
The length of rectangle W is

Breadth of rectangle S
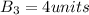
Therefore,
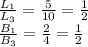
It means ratio of lengths of quadrilateral Q and W is equal to the ratio of their breaths. Therefore W and Q are said to be similar not congruent as they have different lengths and breadths.
Similarly,
ratio of lengths of quadrilateral S and W is equal to the ratio of their breaths. Therefore S and W are said to be similar not congruent as they have different length and breadth.