Answer:
Raising the highest point of the track to a higher point
Step-by-step explanation:
When the rubber ball starts its motion, from the highest point of the track, it has only gravitational potential energy, given by:
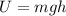
where m is the mass of the ball, g is the gravitational acceleration and h is the height above the ground.
As the ball descends the track, this potential energy is partially converted into kinetic energy, given by:

(where m is the mass and v is the speed)
and partially lost as heat, due to the friction between the surface of the track.
As a consequence, the higher the initial height of the track (h in the formula), the greater will be the kinetic energy gained by the ball. A greater kinetic energy means a larger velocity, which also means that the ball will cover a longer distance before stopping.