Question

Triangle QRS has vertices Q(8, –6), R(10, 5), and S(–3, 3). What are the coordinates of the vertices of the image of the triangle after a translation of T–7.6, 4.3(x, y)? Q′ = R′ = S′ =

Answer 1

Q'= (0.4, -1.7)
R'= (2.4, 9.3)
S'= (-10.6, 7.3)

Answer 2

The coordinates of the vertices of the image of the triangle after a translation are Q'(0.4,-1.7), R'(2.4,9.3) and S'(-10.6,7.3).

Explanation:

It is given that triangle QRS has vertices Q(8, –6), R(10, 5), and S(–3, 3).

Triangle QRS translated by rule T–7.6, 4.3(x, y). This rule is defined as

`(x,y)\rightarrow (x-7.6,y+4.3)`

The coordinates of the vertices of the image of the triangle after a translation are

`Q(8,-6)\rightarrow Q'(8-7.6,-6+4.3)=Q'(0.4,-1.7)`

`R(10,5)\rightarrow R'(10-7.6,5+4.3)=R'(2.4,9.3)`

`S(-3,3)\rightarrow S'(-3-7.6,3+4.3)=S'(-10.6,7.3)`

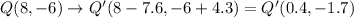
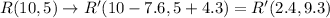
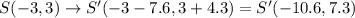
Therefore the coordinates of the vertices of the image of the triangle after a translation are Q'(0.4,-1.7), R'(2.4,9.3) and S'(-10.6,7.3).