Answer:

Explanation:
You can use the quadratic formula to determine if a quadratic equation has real or imaginary solutions. The quadratic formula is:
 . What really matters in this case is the discriminant, which is the stuff under the radical which is:
. What really matters in this case is the discriminant, which is the stuff under the radical which is:
 . This is because the solutions are only imaginary, if the discriminant is negative, because then you would be taking the square root of a negative number. So let's look through each example:
. This is because the solutions are only imaginary, if the discriminant is negative, because then you would be taking the square root of a negative number. So let's look through each example:
 ; a=25, b=20, c-4:
; a=25, b=20, c-4:
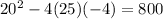 . By examining this one example, it's important to note, if you have one negative number as a or c, then it cancels out the negative sign in the -4, and it becomes positive. So let's look at examples where a or c doesn't equal a negative number OR both a and c equal negative, that way they cancel out and over -4ac is still negative.
. By examining this one example, it's important to note, if you have one negative number as a or c, then it cancels out the negative sign in the -4, and it becomes positive. So let's look at examples where a or c doesn't equal a negative number OR both a and c equal negative, that way they cancel out and over -4ac is still negative.
 ; a=25, b=-10, c=4. In this case both a and c are positive so -4ac will remain negative. This gives you:
; a=25, b=-10, c=4. In this case both a and c are positive so -4ac will remain negative. This gives you:
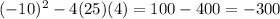 . So this has a negative discriminant meaning it will have no real solution but rather imaginary solutions
. So this has a negative discriminant meaning it will have no real solution but rather imaginary solutions