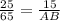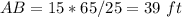Question

Please Help

Kayla wants to find the width, AB, of a river. She walks along the edge of the river 65 ft and marks point C.
Then she walks 25 ft further and marks point D. She turns 90° and walks until her location, point A, and point
C are collinear. She marks point E at this location, as shown.


(a) Can Kayla conclude that Δ and Δ are similar? Why or why not?

(b) Suppose DE = 15 ft. What can Kayla conclude about the width of the river?

Answer 1

Part a) Triangles ABC and CDE are similar by AAA

Part b) The width of the river is
`39\ ft`

Explanation:

Part a)

we know that

If two figures are similar, then the ratio of its corresponding sides is equal and its corresponding angles are congruent

In this problem , triangles ABC and CDE are similar by AAA, because its corresponding angles are congruent

so

`m<DCE=m<ACB`

`m<EDC=m<CBA`

`m<DEC=m<CAB`

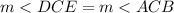
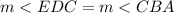
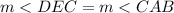
Part b)

Remember that

If two figures are similar, then the ratio of its corresponding sides is equal and is called the scale factor

so

`(DC)/(CB)=(DE)/(AB)`

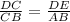
substitute the values and solve for AB (the width of the river)

`(25)/(65)=(15)/(AB)`

`AB=15*65/25=39\ ft`