Question

An object is propelled upward from the top of a 300 foot building. The path that the object takes as it falls to the ground can be modeled by h = 16t^2 + 80t + 800 where t is the time (in seconds) and h is the corresponding height (in feet) of the object. How long does it take the object to hit the ground?

Answer 1

As per the statement:

The path that the object takes as it falls to the ground can be modeled by:

h =-16t^2 + 80t + 300

where

h is the height of the objects and

t is the time (in seconds)

At t = 0 , h = 300 ft

When the objects hit the ground, h = 0

then;

-16t^2+80t+300=0

For a quadratic equation: ax^2+bx+c=0 ......[1]

the solution for the equation is given by:

`x = (-b\pm √(b^2-4ac))/(2a)`

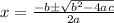
On comparing the given equation with [1] we have;

a = -16 ,b = 80 and c = 300

then;

`t= (-80\pm √((80)^2-4(-16)(300)))/(2(-16))`

`t= (-80\pm √(6400+19200))/(-32)`

`t= (-80\pm √(25600))/(-32)`

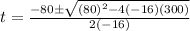
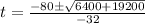
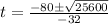
Simplify:

`t = -(5)/(2)` = -2.5 sec and
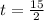`t = (15)/(2)` = 7.5 sec

Time can't be in negative;

therefore, the time it took the object to hit the ground is 7.5 sec