Question

The graph of a quadratic function y = ax^2 + bx + c is shown. Tell whether the discriminant of ax^2 + bx + c = 0 is positive negative, or zero.

Answer 1

The discriminant is equal to 0.

Explanation:

The graph shows the parabola with branches go down in negative y-direction. This gives you that a<0.

Since parabola has one x-intercept, then the equation
`ax^2+bx+c=0` has two equal solutions
`x_1=x_2` and the discriminant is equal to 0.

Answer 2

Answer: Zero

Explanation:

1. By definition: if the discriminant is negative, the quadratic equation does not have real solutions, it has two imaginary solutions. If the discriminant is zero the quadratic has one solution. If the discriminant is positive, the quadratic equation has two distinct solutions.

2. You can see that the function touches the x-axis, this only happen when the solution of the function is even
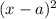`(x-a)^(2)`. Then, the function has only one solution. The discriminant is zero.