Question

Drag an expression or phrase to each box to complete the proof.

Answer 1

<4 ≅ <1, 3 <≅ 2 Corresponding angles postulate

ΔACE ≈ ΔBCD Angle angle similarity postulate

(CB + BA)/CB = (CD + DE)/CD Substitution property of equality

Answer 2

1st blank: ∠4≅∠1 and ∠3≅∠2

2nd blank: Angle-Angle similarity postulate

3rd blank: Substitution property of equality.

Explanation:

Consider the provided figure.

Statement Reason

ΔACE, BD║AE Given

∠4≅∠1 and ∠3≅∠2 Corresponding angle postulate

ΔACE
`\sim` ΔBCD Angle-Angle similarity postulate

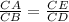`(CA)/(CB)=(CE)/(CD)` Definition of similar triangles

CA=CB+BA and CE=CD+DE Segment addition postulate

`(CB+BA)/(CB)=(CD+DE)/(CD)` Substitution property of equality.

`(CB)/(CB)+(BA)/(CB)=(CD)/(CD)+(DE)/(CD)` Addition of fractions.

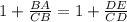`1+(BA)/(CB)=1+(DE)/(CD)` Simplification of fractions

`(BA)/(CB)=(DE)/(CD)` Subtraction property of equality.