Answer:
23m, at t=2s
Explanation:
Putting the physics part aside, you can rewrite the height function as
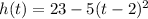 . Now you have a fixed value, 23, and you're subtracting to it a quantity that, being a square, is at least zero. The maximum value of that sum you have when you're not subtracting anything, that is 23. That happens when
. Now you have a fixed value, 23, and you're subtracting to it a quantity that, being a square, is at least zero. The maximum value of that sum you have when you're not subtracting anything, that is 23. That happens when
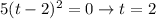
Alternatively, if you know how to determine the velocity from that kind of motion, you can find out the speed, and the highest altitude you reach when the speed is zero, which leads to a bunch of calculation for the same result.