Question

An acorn falls from the branch of a tree to the ground 25 feet below. The distance, S, that the acorn is from the ground as it falls is represented by the equation S(t) = –16t2 + 25, where t is the number of seconds. For which interval of time is the acorn moving through the air?

A.0
B.0
C.T>5/4
D.-5/4

Answer 1

b

Explanation:

got it on edge

Answer 2

`0<t<(5)/(4)`

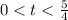
Explanation:

We are given that,

The fall of the acorn is represented by the function,

`S(t) =-16t^2+25`

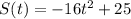
Equating S(t) to 0 gives,

`0=-16t^2+25`

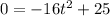
i.e.
`16^2=25`

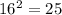
i.e.
`t^(2)=(25)/(16)`

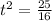
i.e.
`t=\pm (5)/(4)`

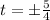
Since, time cannot be negative.

So,
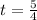`t=(5)/(4)` i.e. 1.25 seconds is the time when the acorn will reach the ground.

Thus, from the figure below, we see that,

The interval in which the acorn is in the moving air is
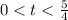`0<t<(5)/(4)`.