Question

Kent invested $5000 in a retirement plan. He allocated x dollars of the money to a bond account that earns 4% interest per year and the rest to a traditional account that earns 5% interest per year. A. Write an expression that represents the amount of money invested in the traditional account. B. Write a polynomial model in simplest form for the total amount of money T Kent has invested after one year. (Hint: Each account has A + IA dollars, where A is the original amount in the account and I is its interest rate.) c. If Kent put $500 in the bond account, how much money does he have in his retirement plan after one year?

Answer 1

a. 5000 - x

b. T=5250 - 0.01x

c. $5245

Answer 2

Answer: The answers are given below.

Step-by-step explanation: Given in the question that Kent invested $5000 in a retirement plan. He allocated x dollars of the money to a bond account that earns 4% interest per year and the rest to a traditional account that earns 5% interest per year.

(A) Let, '$y' be the amount of money Kent invested in the traditional account, then we have

`y=5000-x.`

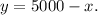
This is the required expression.

(B) After 1 year, total money in the bond account will be

`M_b=x+(1* 4* x)/(100)=x+(x)/(25)=(26)/(25)x,`

and amount of money in the traditional account will be

`M_t=(5000-x)+(1* 5* (5000-x))/(1000)=(5000-x)+(5000-x)/(200)\\\\\\\Rightarrow M_t=(201(5000-x))/(200).`

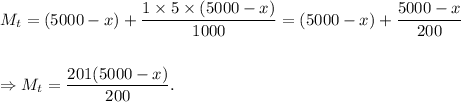
Therefore, total amount invested after 1 year will be

`M=M_b+M_t=(26)/(25)x+(201(5000-x))/(200)=(208x+1005000-201x)/(200)\\\\\\\Rightarrow M=(7x+1005000)/(200).`

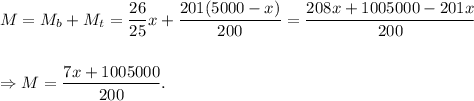
(C) If x = $500, then we have

`M=(7* 500+1005000)/(200)=(1008500)/(200)=(10085)/(2)=5042.5.`

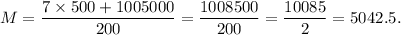
Thus, Kent will have $5042.5 in his retirement plan after 1 year.