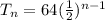Answer:
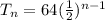 defines the relationship.
defines the relationship.
Explanation:
In a large single elimination basketball tournament, the first round of the play begins with 64 teams.
In each successive round, the number of teams remaining in the tournament is reduced by half.
So the sequence formed will be 64, 32, 16, 8........
Now we have to find the relationship.
In the sequence there is a common ratio of
 in each successive term
in each successive term
r =
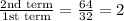
Since explicit formula of an exponential sequence is
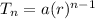
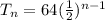
Therefore, equation representing the relationship will be