Answer:
Cost of per session the average rate is $45.
Explanation:
It is given that a gym membership with two personal training session cost $125, while gym membership with five personal training sessions cost $260.
It is required to find what is the cost per session.
Step 1 of 1
It is given that a gym membership with two personal training session cost $125, while gym membership with five personal training sessions cost $260.
To find the cost of per session calculate the average rate.
Now let $f(x)$ be the cost per session use the for the average rate of change, and the input value is the number of personal traings x.
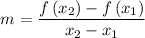
Now substitute, $125 for
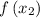 , 260 for
, 260 for
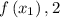 for
for
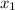 and 5 for
and 5 for
 then,
then,

Cost of per session the average rate is $45.