Question

3. Try It #3 Write the point-slope form of an equation of a line with a slope of -2 that passes through the point (-2,2). Then rewrite it in the slope-intercept form.

Answer 1

Point-slope form of equation given as
`$y-2=-2(x+2)$`.

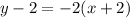Slope-intercept form of equation is given as
`$y=-2 x-2$`.

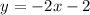Explanation:

In the question, it is given that the slope of a line is -2 and it passes from (-2,2).

It is asked to write the point-slope form of the equation and rewrite it as slope-intercept form.

To do so, first find the values which are given in the question and put it in the formula of point-slope form. Simplify the equation to rewrite as slope-intercept form.

Step 1 of 2

Passing point of the line is (-2,2).

Hence,
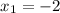`$x_(1)=-2$` and

`$$y_(1)=2 \text {. }$$`

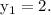
Also, the slope of the line is -2.

Hence, m=-2

Substitute the above values in point-slope form of equation given by
`$y-y_(1)=m\left(x-x_(1)\right)$`

`$$\begin{aligned}&y-y_(1)=m\left(x-x_(1)\right) \\&y-2=-2(x-(-2) \\&y-2=-2(x+2)\end{aligned}$$`

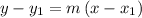
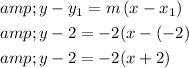
Hence, point-slope form of equation given as y-2=-2(x+2).

Step 2 of 2

Solve y-2=-2(x+2) to write it as slope-intercept form given by y=mx+c.

`$$\begin{aligned}&y-2=-2(x+2) \\&y-2=-2 x-4 \\&y=-2 x-4+2 \\&y=-2 x-2\end{aligned}$$`

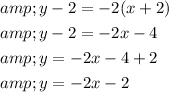
Hence, slope-intercept form of equation is given as y=-2x-2.