For this case, they ask us to determine which of the improper fractions (Numerator is greater than or equal to the denominator) given is not equivalent to "3".
Option A:
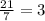
Three times the number "7" gives "21".
Option B:
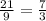
Note that if we divide the numerator and denominator by "3" we get the fraction \ frac {7} {3} other than the integer "3".
Option C:
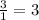
Option D:
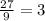
Three times the number "9" gives "27"
Thus, the fraction that is not equivalent to "3" is:
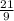
Answer:
Option B