Question

31. For the following exercises, given each set of information, find a linear equation satisfying the conditions, if possible.

31. f(-1) = 4 and f(5) = 1

Answer 1

The required linear equation satisfying the given conditions f(-1)=4 and f(5)=1 is
`$y=(-1)/(2) x+(7)/(2)$`

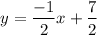
Explanation:

It is given that f(-1)=4 and f(5)=1.

It is required to find out a linear equation satisfying the conditions f(-1)=4

and f(5)=1. linear equation of the line in the form

`$$\left(y-y_(2)\right)=m\left(x-x_(2)\right)$$`

Step 1 of 4

Observe, f(-1)=4 gives the point (-1,4)

And f(5)=1 gives the point (5,1).

This means that the function f(x) satisfies the points (-1,4) and (5,1).

Step 2 of 4

Now find out the slope of a line passing through the points (-1,4) and (5,1),

`$$\begin{aligned}&m=(y_(2)-y_(1))/(x_(2)-x_(1)) \\&m=(1-4)/(5-(-1)) \\&m=(-3)/(5+1) \\&m=(-3)/(6) \\&m=(-1)/(2)\end{aligned}$$`

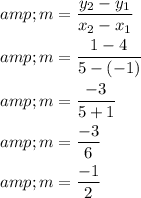
Step 3 of 4

Now use the slope
`$m=(-1)/(2)$` and use one of the two given points and write the equation in point-slope form:

`$(y-1)=(-1)/(2)(x-5)$`

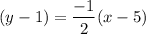
Distribute
`$(-1)/(2)$`,

`$y-1=(-1)/(2) x+(5)/(2)$`

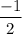Step 4 of 4

This linear function can be written in the slope-intercept form by adding 1 on both sides,

`$$\begin{aligned}&y-1+1=(-1)/(2) x+(5)/(2)+1 \\&y=(-1)/(2) x+(5)/(2)+(2)/(2) \\&y=(-1)/(2) x+(7)/(2)\end{aligned}$$`

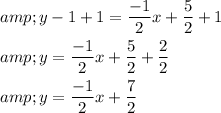
So, this is the required linear equation.