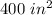Question

What is the area of this figure

if you can't tell im not smart please help

Answer 1

the area is might be 26

Answer 2

For this case, we must find the area of the figure composed of a parallelogram and a right triangle.

Let:

a: Base

h: Height

The area of the triangle is given by:

`A = \frac {b * h} {2}\\A = \frac {20 * 8} {2}\\A = \frac {160} {2}\\A = 80 \ in ^ 2`

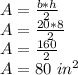
The area of the parallelogram is given by:

`A = b.h\\A = 20 * 16\\A = 320 \ in ^ 2`

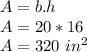
The total area is given by the sum of the areas:

`At = (80 + 320) in ^ 2\\At = 400 \ in ^ 2`

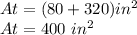
Answer:

`400 \ in ^ 2`