Answer:
the new volume of the prism is
 times the original volume
times the original volume
Explanation:
we know that
If two figures are similar, then the scale factor elevated to the cube is equal to the ratio of its volumes
Let
z------> the scale factor
x------> the volume of the dilated prism
y------> the volume of the original prism
so
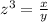
In this problem we have
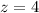
substitute

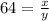

that means-----> the new volume of the prism is
 times the original volume
times the original volume