Question

Which triangle is similar to △ABC if sin(A)=1/4, cos(A)=√15/4, and tan(A)=1/√15?

Answer 1

Explanation:

Answer 2

(D)

Explanation:

The given triangle ABC has sinA=
`(1)/(4)`, cosA=
`(√(15))/(4)` and tanA=
`(1)/(√(15))`.

From the given options, option D is correct because, SinX=
`(6)/(24)=(1)/(4)`,

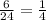cosX=
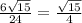`(6√(15))/(24)=(√(15))/(4)` and

tanX=
`(6)/(6√(15))=(1)/(√(15))`

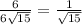
Since, the values of sin, cos and tan of triangle XYZ are similar to teh values of triangle ABC, therefore triangle XYZ is similar to triangle ABC.