Question

Jack’s shipping company has to transport boxes of industrial nuts and industrial bolts to its clients. Each box of industrial nuts weighs 100 kilograms, and each box of industrial bolts weighs 200 kilograms. The maximum weight the company’s cargo container can hold is 24,000 kilograms. To minimize transportation costs, the company needs to transport a minimum of 160 boxes per cargo container.

Let x be the number of boxes with industrial nuts, and y be the number of boxes with industrial bolts.

Which of the following graphs represents the situation with the solution region shaded?

Graph A
Graph B
Graph C
Graph D
Cannot be determined from the given information

Answer 1

The correct option is B.

Explanation:

Let the boxes of nuts be x and the boxes of bolts by y.

The weight of nut box is 100 kg and the weight of bolt box is 200 kg.The maximum weight the company’s cargo container can hold is 24,000 kilograms.

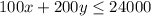
`100x+200y\leq 24000`

`x+2y\leq 240` .... (1)

The company needs to transport a minimum of 160 boxes per cargo container.

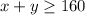`x+y\geq 160` .... (2)

Plot the above inequalities on the coordinate plane.

The related equations are

`x+2y=240` .... (3)

`x+y=160` .... (4)

The x-intercept of equation 3 is (240,0) and the y-intercept is (0,120).

The x-intercept of equation 4 is (160,0) and the y-intercept is (0,160).

Check the inequality by (0,0).

`0+2(0)\leq 240`

`0\leq 240`

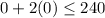
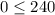
This statement is true, therefore the shaded region of inequality (1) contains (0,0).

`x+y\geq 160`

`0+0\geq 160`\

`0\geq 160`

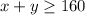
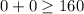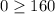
This statement is false, therefore the shaded region of inequality (2) does not contains (0,0).

Therefore graph (2) represents the solution and option B is correct.