Answer:
Option C is correct.
She is not correct as she use the wrong y intercepts when graphing the equations.
Explanation:
Given the system of equations:
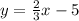 .....[1]
.....[1]
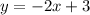 . .....[2]
. .....[2]
equate these two equations we get;
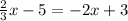
Add 5 to both sides we get;
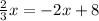
Add 2x to both sides we have;
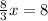
Multiply both sides by 3 we get;
8x = 24
Divide both sides by 8 we get;
x = 3
Substitute the value of x in [2] we have;
y = -2(3)+3 = -6+3
Simplify:
y = -3
Therefore, the correct solution for this given system of equation is (3, -3)
You can see the graph of these equations below.